Factorizing algebraic expressions is a fundamental skill in mathematics that opens the door to solving complex equations and understanding the underlying structure of algebraic forms. Whether you're a student grappling with your first algebra course or an advanced learner looking to sharpen your skills, mastering this technique is essential. By breaking down expressions into their simplest components, you not only enhance your problem-solving abilities but also gain deeper insights into the relationships among numbers and variables.
In the world of mathematics, factorization is akin to the process of simplifying a recipe; just as a chef might break down ingredients to their basic forms, mathematicians take complex expressions and unravel them into simpler factors. This process not only makes calculations easier but also plays a crucial role in various mathematical applications, from solving equations to graphing functions. With the right approach, anyone can learn to factorize algebraic expressions with confidence and precision.
As we delve deeper into this topic, we'll explore the various methods of factorization, practical examples, and tips for mastering this essential skill. Whether you are preparing for an exam or just looking to improve your math skills, understanding how to factorize algebraic expressions will serve as a crucial tool in your mathematical toolkit.
What is Factorization in Algebra?
Factorization refers to the process of decomposing an algebraic expression into a product of simpler expressions, known as factors. This method allows mathematicians to rewrite the expression in a form that simplifies calculations and reveals critical information about the original expression. Understanding how to factorize algebraic expressions is a vital skill for solving equations, simplifying fractions, and working with polynomials.
Why is Factorizing Algebraic Expressions Important?
Factorizing algebraic expressions is crucial for several reasons:
- It simplifies complex expressions, making calculations more manageable.
- It helps solve equations by setting factors equal to zero.
- It allows for easier manipulation of algebraic expressions in various mathematical contexts.
- Understanding factorization lays the groundwork for more advanced topics in algebra and calculus.
How Do You Factorize Algebraic Expressions?
Factorizing algebraic expressions involves several techniques, including:
- Common Factor Extraction: Identifying and factoring out the greatest common factor from the expression.
- Grouping: Rearranging terms into groups and factoring them separately.
- Using Special Products: Recognizing patterns such as the difference of squares or perfect square trinomials.
- Quadratic Factorization: Applying methods to factor quadratic expressions of the form ax² + bx + c.
What Are the Steps to Factorize Algebraic Expressions?
To factorize algebraic expressions effectively, follow these steps:
- Identify Common Factors: Look for the greatest common factor in the expression.
- Rearrange Terms: If applicable, rearrange the terms to facilitate grouping.
- Group Terms: Group terms that share common factors.
- Factor Out Common Elements: Factor out the common elements from each group.
- Combine Factors: Write the expression as a product of its factors.
Can You Provide Examples of Factorizing Algebraic Expressions?
Let's look at a few examples:
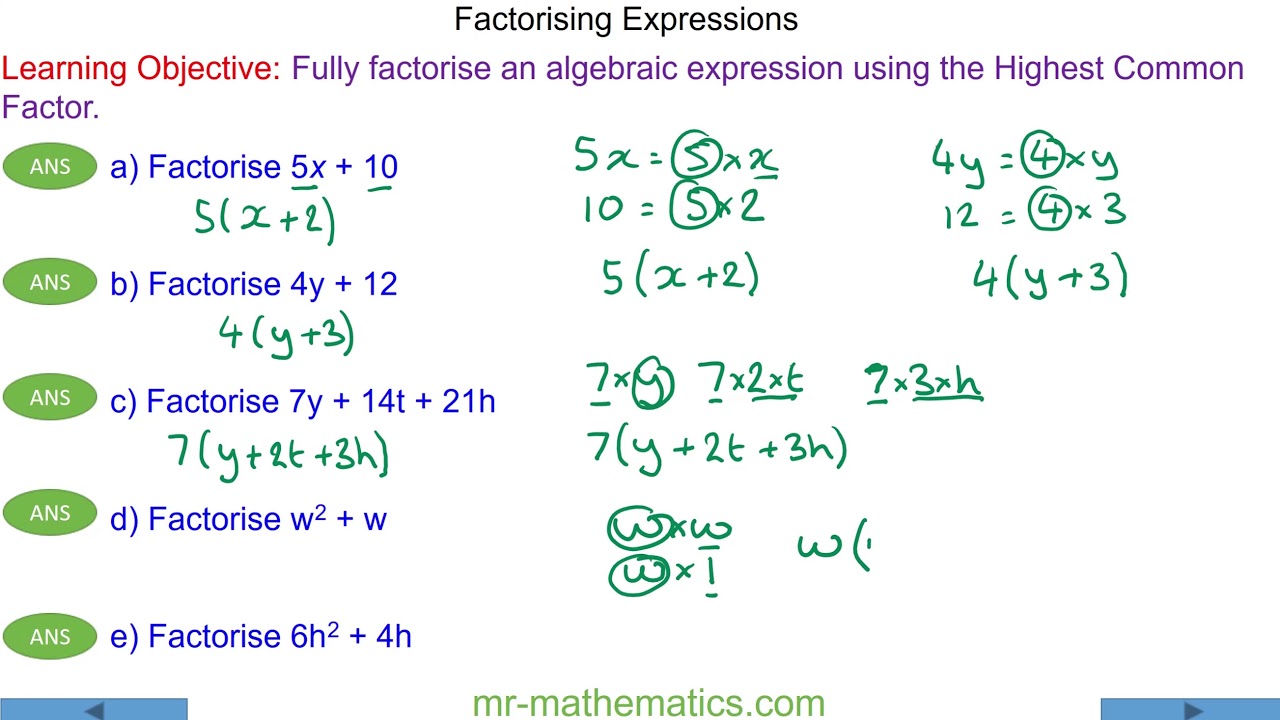
Example 1: Factor the expression 6x² + 9x.
Step 1: Identify the common factor, which is 3x.
Step 2: Rewrite the expression as 3x(2x + 3).
Example 2: Factor the quadratic expression x² + 5x + 6.
Step 1: Look for two numbers that multiply to 6 and add to 5, which are 2 and 3.
Step 2: Rewrite the expression as (x + 2)(x + 3).
What Are Some Common Mistakes When Factorizing Algebraic Expressions?
While factorizing algebraic expressions, students often make several common mistakes, including:
- Failing to identify the greatest common factor.
- Overlooking special products, such as the difference of squares.
- Forgetting to check their work by expanding to ensure the factorization is correct.
- Neglecting to factor completely, leaving some factors unaccounted for.
How Can You Practice Factorizing Algebraic Expressions?
To become proficient at factorizing algebraic expressions, consistent practice is essential. Here are a few tips for effective practice:
- Work through practice problems in textbooks or online resources.
- Join study groups to collaborate and learn from peers.
- Use educational apps and websites that offer interactive factorization exercises.
- Teach the concept to someone else to reinforce your understanding.
What Resources Are Available for Learning Factorization?
There are numerous resources available for those looking to master the art of factorizing algebraic expressions:
- Textbooks: Many algebra textbooks provide thorough explanations and ample practice problems.
- Online Courses: Websites like Khan Academy offer free lessons on factorization and other algebra topics.
- Tutoring: Consider hiring a tutor for personalized instruction and guidance.
- Video Tutorials: Platforms like YouTube have countless tutorials that visually demonstrate factorization techniques.
In conclusion, mastering how to factorize algebraic expressions is a vital skill that can significantly enhance your mathematical abilities. With regular practice and the right resources, anyone can become proficient in this essential technique, paving the way for future success in algebra and beyond.
The Legendary Journey Of Ronaldinho With The Brazil Soccer Team
Lara Fabian: The Heartfelt Journey Of "Broken Vow"
Discovering Treasures At The Harrisburg Flea Market