The arithmetic series formula is a powerful mathematical tool that enables individuals to easily calculate the sum of a series of numbers that follow a specific pattern. This formula is essential in various fields, including finance, physics, and computer science, making it a vital concept for students, educators, and professionals alike. Whether you’re attempting to understand basic arithmetic or delving into more complex mathematical theories, comprehending the arithmetic series formula can provide you with a clearer perspective on how numbers interact in a sequence.
At its core, an arithmetic series is simply the sum of the terms in an arithmetic sequence. An arithmetic sequence is one in which each term after the first is created by adding a constant difference to the previous term. This constant difference plays a crucial role in the arithmetic series formula, as it determines the nature of the series itself. Understanding this concept not only enhances mathematical skills but also sharpens critical thinking and problem-solving abilities.
In this article, we will explore the intricacies of the arithmetic series formula, answer common questions, and provide practical examples to illustrate its application. Whether you are a student looking to boost your math skills or a professional seeking to apply these concepts in real-life scenarios, you’ll find valuable insights and guidelines on how to effectively use the arithmetic series formula.
What is the Arithmetic Series Formula?
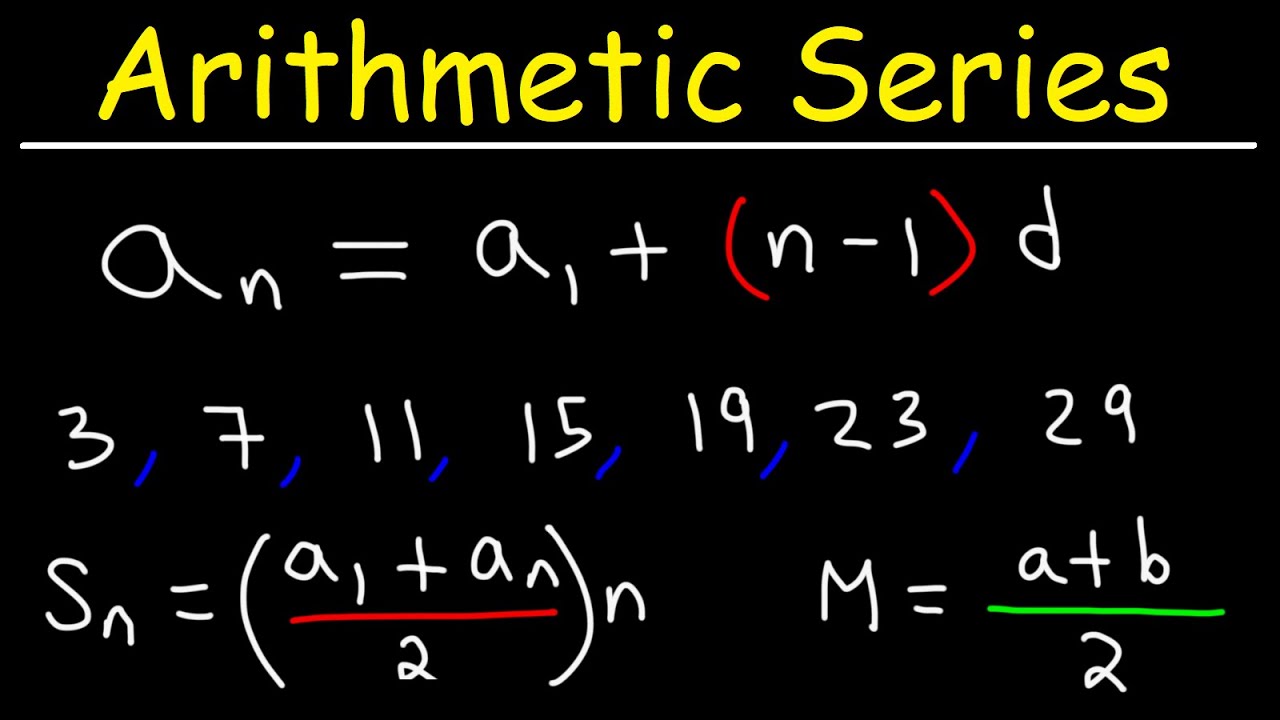
The arithmetic series formula is used to find the sum of the first \( n \) terms of an arithmetic series. It is expressed as:
S_n = \frac{n}{2} \times (a + l)
Where:
- S_n = Sum of the first \( n \) terms
- n = Number of terms
- a = First term
- l = Last term
How Do You Derive the Arithmetic Series Formula?
To derive the arithmetic series formula, consider an arithmetic series with \( n \) terms. When you write the series forwards and backwards and then add them together, you notice that each pair of terms sums to the same total. This observation is key in deriving the formula. By combining the two series, you can simplify and isolate \( S_n \) to arrive at the formula mentioned earlier.
What Are Some Examples of Arithmetic Series?
Arithmetic series can be found in various real-life situations. Here are a few examples:
- Calculating total payments in a loan with fixed monthly payments.
- Summing scores in a series of games where each score increases by a fixed amount.
- Determining the total distance traveled in a series of trips where each trip is a consistent distance longer than the previous one.
How Do You Use the Arithmetic Series Formula in Real Life?
The arithmetic series formula is not just a theoretical concept; it has practical applications in several fields. For instance, in finance, it can help evaluate loan repayments, while in statistics, it can assist in analyzing data trends. Here’s how it can be applied:
- Identify the first term of the series.
- Determine the number of terms you wish to sum.
- Calculate the last term using the formula of the arithmetic sequence.
- Apply the arithmetic series formula to find the total sum.
What Are the Common Mistakes When Using the Arithmetic Series Formula?
Even experienced individuals can make errors when applying the arithmetic series formula. Here are some common pitfalls to watch out for:
- Forgetting to identify the correct first term or last term.
- Miscalculating the number of terms in the series.
- Confusing arithmetic sequences with geometric sequences.
How Can You Practice Using the Arithmetic Series Formula?
To master the arithmetic series formula, regular practice is essential. Here are a few tips on how to practice effectively:
- Work on exercises that involve both simple and complex arithmetic series.
- Use online resources and math tools that provide practice problems.
- Collaborate with peers or tutors to challenge each other with problems.
What Are the Benefits of Understanding the Arithmetic Series Formula?
Grasping the arithmetic series formula has several advantages. Understanding this concept can:
- Enhance problem-solving skills and mathematical reasoning.
- Provide a solid foundation for more advanced mathematical concepts.
- Improve analytical skills useful in various professional fields.
Conclusion: Why is the Arithmetic Series Formula Important?
The arithmetic series formula is a fundamental concept that serves as a building block for many mathematical applications. By understanding and applying this formula, individuals can unlock a world of possibilities in both academic and professional settings. Whether you’re a student preparing for exams or a professional tackling complex calculations, mastering the arithmetic series formula is an invaluable asset that can enhance your mathematical journey.
Exploring The World Of Eddie Murphy's Donkey: A Journey Into Animation And Laughter
Understanding The Term "Based": A Cultural Exploration
Discovering The Smallest Person In The World